Variational Quantum Algorithms for Nonlinear Partial Differential Equations and Quantum Physics on Complex Graphs
P4a. Tensor networks for partial differential equations:

Foto: UHH/Jaksch
Many important partial differential equations can be solved as nonlinear optimization problems on quantum computers or with quantum-inspired algorithms. In this project, we will investigate and quantify the quantum advantages of studying the Gross-Pitaevskii equation via quantum-inspired methods. The Gross-Pitaevskii equation is a paradigm example of a nonlinear mean-field theory that accurately describes many interaction effects arising in Bose-Einstein condensates at ultra-low temperatures. Despite utilizing a mean-field approximation, this equation is challenging to solve. Here, we apply tensor network methods to study particularly challenging situations where ultracold atoms are stored in quasiperiodic optical lattices. We started by investigating the ground-state properties of a weakly interacting Bose gas confined in a quasiperiodic lattice and specifically the 1D Aubry-André Model. We have observed the occurrence of one-particle and many-body localization, known as Anderson localization as well as a Bose Glass phase. Our next steps are to extend our research to two-dimensional quasiperiodic systems and explore large-sized systems that are only numerically accessible through the power of tensor network methods. The results of this study will enable us to make quantum computers useful for problems beyond quantum physics and explore new industry-related research applications.
P4b. Quantum physics on complex graphs:
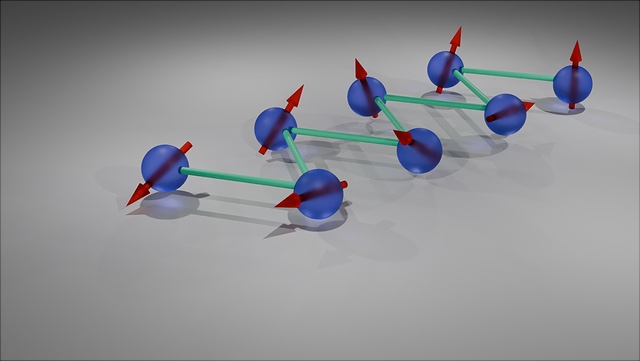
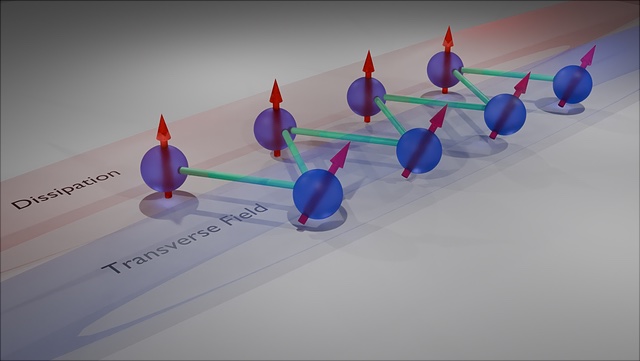
Foto: UHH/Jaksch
Here, we investigate the dynamical evolution of open many-body quantum systems whose symmetries prevent them from thermalizing. Such dissipation-induced dynamical symmetries can lead to long-lived metastable ordered quantum phases and persistent oscillations. Specifically, we have been studying a spin ladder where the upper leg has contact with the bath going under the dissipation process and the other leg is under the influence of the transverse field isolated from the bath. We found that by tuning the properties of dissipation and transverse field, the emergence of synchronization takes place. The degree of synchronization depends on the interaction between the system and the bath. Utilizing modern experimental tools for controlling, manipulating, and steering ultracold atoms at the quantum level, we expect that a wide range of non-Hermitian quantum dynamics can be engineered and controlled in these systems. Our work aims to make the exciting field of non-Hermitian quantum physics, which is currently mainly a theoretical study, accessible to experimental realization.
